Visualization of Selected Sangaku Problem as Didactical Phenomenon in GeoGebra

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102-08, May 2021.

 Visualization of Selected Sangaku Problem
 as Didactical Phenomenon in GeoGebra
 Dusan Vallo

Department of Mathematics, Faculty of Natural Sciences, Constantine the Philosopher University in Nitra, Tr.
 A. Hlinku 1, 949 01 Nitra, Slovakia

 Abstract - In this article, we will focus on 2. Dynamical Geometry Software in Teaching
visualization solutions within specific geometric and Education
problem of the category Sangaku - historical
mathematical problems from Japan. We also highlight The first visionary who predicted the rise of
didactical advantages of experimental activities in the
 computers and their significant use in teaching and
teaching of mathematics and the role of the
visualization via using dynamical geometry software also in mathematics was Seymour Papert in his book
GeoGebra. [1]. From the beginning, mathematical software was
 covered only specific areas of mathematics teaching.
 Keywords - sangaku, GeoGebra, CAS environment,
 This initial specialization proved to be problematic
visualization, arithmetic sequence, Apollonius’ net.
 [2].
 According to [3] educational software is a tool that
1. Introduction
 helps to experiment and manipulate objects in
 constructions, to discover relationships or
 In this article, we focus on a visualization of a
 regularities, and supports research.
specific mathematical task that belongs to a group of
 It turns out that currently available dynamic
historical Japanese geometric problems called
 geometric software, such as GeoGebra, is a good
sangaku.
 example of such educational software.
 We use the dynamic geometric software
 Dynamic geometric software makes it possible to
GeoGebra, its tools, commands, and environments to
 be used across the entire curriculum. It integrates a
analyze a solution. This approach emphasizes the
 few educational environments and also offers the
importance of interdisciplinary relationships among
 possibility of discovering mathematical content in a
algebra, analytical geometry, and programming.
 form of an experiment.
 It also highlights didactical advantages of
 The role of the experiment is to purposefully
experimental activities in the teaching of
 acquire experience. For students, this is an
mathematics and the role of visualization.
 irreplaceable way of acquiring knowledge through
 their own activity [4].
 The integration of the dynamic geometric software
DOI: 10.18421/TEM102-08 into the teaching of mathematics allows an analysis
https://doi.org/10.18421/TEM102-08 of a mathematical problem from several perspectives.
Corresponding author: Dusan Vallo, It has tools on how to visualize all these perspectives,
Department of Mathematics, Faculty of Natural Sciences, too.
Constantine the Philosopher University in Nitra, Nitra, Visualization is an important element in
Slovakia. mathematical thinking and has an important role in
Email: dvallo@ukf.sk discovering relationships, their transfer to learners,
 and communication in mathematics generally [5].
Received: 11 January 2021.
Revised: 17 March 2021.
 3. What is Sangaku?
Accepted: 24 March 2021.
Published: 27 May 2021.
 The term Sangaku denotes wooden tablets that
 © 2021 Dusan Vallo; published by UIKTEN. have been preserved as artifacts in Shinto and
This work is licensed under the Creative Commons Buddhist temples in Japan. These tablets are painted
Attribution‐NonCommercial‐NoDerivs 4.0 License. artworks of high mathematical and cultural value
The article is published with Open Access at from the Edo period (1603-1867) when Japan was
www.temjournal.com isolated from Western influence [6].

 540 TEM Journal – Volume 10 / Number 2 / 2021.

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102‐08, May 2021.

 Sangaku is not a local phenomenon in any part of It holds
Japan. It is a cultural heritage that has been preserved
 312 1. 2 . 3.13
thanks to the military elite of medieval Japan. These
 1.312 2.156 3.104 4.78 6.52 8.39
warriors, called samurai maintained contacts across
 12.26 13.24.
all regions and professed the life philosophy of
"sword and pen". The samurai ran schools in which It leads to systems of equations listed in Table 1.
they practiced martial arts, especially workout with a
long sword. The second attribute of their life was Table 1. System of equation related to Problem 1
focused on literature, and mathematics [7]. , 
 Equations 
 
 26 1
 13 137 162
 312
 1, 312
 26
 156,155
 312 137 149
 12075
 13 1
 26 2 119 167
 13
 156 2 2
 2,156
 26 119 141
 Figure 1. Sangaku (1859) Source: [8] 156
 13 2 2 2
 26
 Each table records a mathematical theorem or 53,50
 104 34 44
geometric problem that is presented to a reader for a 1260
 13 3
 3,104
solution. 26 3
 A special combination of precise mathematical 13 34 57
 104
nature and art makes this phenomenon of Japanese 43 87
 26 4
mathematical culture exceptional. 13 78
 4,78 2 2
 Many of these wooden tablets have been destroyed 26 78 43 61
or not recognized, e.g. one sangaku was found in 13 4 2 2
2005, when a Japanese expert, Fukagawa Hidetoshi, 26 6 19 59
visited the Kumano Temple in Senhoku City. He 13 52 2 2
 6,52
 26 52 19 33
identified a tablet from 1858 as a sangaku describing
 13 6 2 2
a mathematical problem about the storage of balls. 26 8
 In [7] a reader can find this task denoted as 4 22
 13 39
 8,39
Problem 40. It assignment is as follows. 26 39
 4 9
 23,15
 13 8 105
4. Problem of Stored Balls 26 12 1 27
 13 26 2 2
 12,26
 26 26 1 1
 We have M balls stored in two stacks , . First, in 13 12 2 2
 we stack them with 19 balls on the top and m balls on 26 13
 1 12
the bottom. Then in we can stack them with 6 balls on 13 24
 13,24
top and n on the bottom. Find M, m, and n. 26 24
 1 1
 Solution. We solve this problem as a task related to 13 13
arithmetical sequence [9].
 Remark. In [8, p. 138] we find the solutions
 If we label 1, resp. 1 the numbers of rows
 23, 15, and 156, 155.
in , resp. , then it holds that
 They are presented as the only options. The solution
 2 1 19 1 6 ,
 53, 50 has remained unnoticed.
where
 19 , 6 4.1. CAS Visualization in GeoGebra
 due to difference 1 of the arithmetic sequence Solving a system of two linear equations with two
in both cases , . variables , without the usage of ICT is an easy but
From (1) we derive that lengthy process. We use CAS environment of
 26 13 312. Geogebra to find all solutions of the systems of the
 equations.
We find a solution , ∈ and therefore we
use a numerical decomposition of number 312.

TEM Journal – Volume 10 / Number 2 / 2021. 541

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102‐08, May 2021.

 First, using tool Slider in Graphics window we put
 , ,
parameters , , , , ∈ such that ∈ ⟨1, ⟩,
 ∈ ⟨1, ⟩, ∈ , 0 and is auxiliary counter. , ,
 The parameter , resp. represents the number of
the balls on the top of the stack , resp. stack , is for both cases of the stacks , .
radius of the ball and, , are freely adjustable The suitable values of , we set up in Graphic
positive integers. window via sliders , manually.
 These general input parameters lead to an equation In Fig. 2 is calculated
 1 , 34, 44, 53, 50, 1260.
Where The flexibility of this CAS’ approach allows easy
 to verify the results and it also encourages students to
 1 .
 experiment.
In Input bar we apply the command Some of these results by changed inputs are listed
 DivisorsList( ) in Table 2.

 to produce a list of all divisors of the number . The Table 2. Some experimental solutions
command is in the form
 
 p1=DivisorsList( 1 ), 6 8 1 6 21 2 5
 18 20 7 12 57 2 5
which can be repeated v 1st row of CAS window due
 18 45 7 42 882 27 35
to some monitoring.
 38 56 5 42 893 18 37
 In Graphic window we set up the auxiliary counter 37 39 4 15 114 2 11
 such that
 ∈ ⟨1, 1 ⟩, 4.2. Graphics Visualization
where Divisors 1 yields the Interesting visualization gives a connection to the
number of all positive divisors of the number . graphs interpretation of the equation. The solutions
 , , ∈ can be also
Now, in CAS window we twice apply the command estimated like grid points lying on a hyperbola in the
 Solve(, ) equation
in the forms 1 .
 Solve ({x+y+a+b+1=p1(j), x-y+a-b=(a-b)*(a+b-
 1)/p1(j)}, {x,y}) The regular solution of the task is restricted to the
 1st quadrant of the Cartesian coordinate system.
and
 Solve ({x+y+a+b+1=(a-b)*(a+b-1)/p1(j), x-y+a-
 b=p1(j)}, {x,y}),

 Figure 2. Solutions in CAS window for 3, 3,104 .
 Figure 3. Visualizations in Graphics window for 
 If we manually change the value of the counter ,
 3, 3,104 via points , 
the software yields all solutions , ∈ 
corresponding to the order of the individual divisors. The intuitive approach on how to find the grid
 In Spreadsheet window we calculate the number of points lying on the given hyperbola manually can be
balls by using the formulas difficult and confusing.

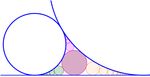
 542 TEM Journal – Volume 10 / Number 2 / 2021.

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102‐08, May 2021.

 Any grid point can visually be a point of 5. Sangaku Inspires
hyperbola, but it need not be true. The student can
verify its position via the implemented tool Relation. In the paper [10] one can find a solution to a
 geometric problem inspired by sangaku (related to
4.3. Stacks Visualization in Graphics Problem 16 in [7], p. 176). The fundamental idea is
 based on the construction of a chain of tangent
 Sangaku is unique due to its visual design. It is a circles.
presentation of the desired solution together with the
 Problem
assignment. Taking into account this fact, we have a
natural requirement to draw the result in the form of Two externally tangent circles , , , 
a picture. have a common tangent line . Find the sequence of
 If we calculate the parameters , evaluated in radii of circles for 3,4,5, … in terms of
CAS system, and then apply the command , , (Fig. 5).
 Sequence(,, , , ) holds

in the form ,
 √ √
S=Sequence(Sequence(((2j + k) r, k r sqrt(3)), j, 0, m if we consider the chain linking in Fig. 5 (the circles
- k, 1), k, 1, m - a + 1, 1). proceed to the left of the circle ) [10].
 It allows us to drawing centers of circles; By analogy, the formula
representing the balls in stack . We construct the 1 2
circles with the command 
 √ √ 
Sequence(Sequence(Circle(S(j, k), r), j, 1, m - k + 1), determines the radii of the circles proceed to the right
k, 1, m). of the circle .
 The application of these commands constructs the
stack in Graphics environment (Fig. 4)

 Figure 4. The stacks , for 4, 1 Figure 5. The problem of chain linking of the circles

 Similarly, the stack can be displayed by using 5.1. Visualization in GeoGebra
C=Sequence(Sequence((-4r - (2j + k) r, k r sqrt(3)), We apply analytical method to the visualization of
j, 0, n - k, 1), k, 1, n - b + 1, 1) the problem.
and The tangent line is in equation 0 and the
Sequence(Sequence(Circle(C(j, k), r), j, 1, n - k + 1),
 centers 0, , 2√ , . The sliders , , in
k, 1, n).
 The –coordinates of the centers of the circles in Graphics window determine the input values of the
the stack are translated by vector value given radii and the number of circles which will be
 displayed.
 ⃗ 4 2 , 0 Using the command Sequence in the form
due to its useful position in the 2nd quadrant. l1=Sequence(((j - 2) / sqrt(R) + 1 / sqrt(r))⁻², j, 3,
 The usage of the commands Sequence requires n)
mastering the basics of programming and it can be we define the radii of the circles in left chain linking
confusing for the students. On the other side, this and the command
kind of visualization enhances the aesthetic
experience of solving the problem. l2= Sequence((2sqrt(R l1(i)), l1(i)), i, 1, n - 2)
 defines the coordinates of the centers.

TEM Journal – Volume 10 / Number 2 / 2021. 543

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102‐08, May 2021.

 Finally, the command ruler and compass, a circle tangent to three given
 circles [11].
 l3=Sequence(Circle(l2(k), l1(k)), k, 1, n - 2)
 We apply this idea to the circles , and .
displays the circles. In [10] the formula
 By analogy, the commands 1
 
l4=Sequence(((j - 2) / sqrt(r) + 1 / sqrt(R))⁻², j, 3, n), 1 1 1
 2 2 2
 l5=Sequence((2sqrt(R r) - 2sqrt(r l4(j)), l4(j)), j, 1, 
 n - 2), is derived. It allows us to construct the chain linking
 l6= Sequence(Circle(l5(j), l4(j)), j, 1, n - 2) of the circles in Fig. 8.
visualize the right chain linking of the circles.

 Figure 8. The extension of the primary solution
 Figure 6. The problem of chain linking of the circles
 In GeoGebra environment we solve this task via the
 The circle is tangent circle to each of the given commands
objects , , and . It is not a unique solution to this
property. The next one is the circle Fig. 7 . l7 = Sequence(1 / (2 (j - 2) sqrt(1 / (r R)) + (j - 2)² (1
 / r + 1 / R)), j, 3, n),
 l8 = Sequence(Circle(O_1, R + l7(j)), j, 1, n - 2),
 l9 = Sequence(Circle(O_2, r + l7(j)), j, 1, n - 2),
 l10= Sequence(Intersect(l9(j), l8(j)), j, 1, n - 2),
 l11= Sequence(Circle(l10(j), l7(j)), j, 1, n - 2).
 This is a different approach. The idea is based on
 the intersections of auxiliary circles defined by the
 commands l8, l9.
 The command l11 uses these intersections points as
 the centers of the circles defined by the command
 l11.
 Completing this set of the circles we obtain some
 interesting filling of the space among three original
 objects , and (Fig. 9).
 Simultaneously it can be solved for , and .
 Figure 7. Tangent circles to the given objects

 It holds true that the radius of the circle is
 1 1
 
 √ √ 
 for .
 The construction of the chain linking of the circles
 , and their tangent line is analogous to case of
described above. Details are left to the reader.

5.2. Extension to Apollonius’ Problem Figure 9. The chain linking as non-linear problem

 The original Apollonius’ problem of the three
circles has dated from antiquity: to construct, with

 544 TEM Journal – Volume 10 / Number 2 / 2021.

TEM Journal. Volume 10, Issue 2, Pages 540‐545, ISSN 2217‐8309, DOI: 10.18421/TEM102‐08, May 2021.

 If we apply the idea of the last construction, then References
we can construct the Apollonius’ net (or Apollonian
gasket) [12]. [1]. Papert, S. (1993). The children's machine: Rethinking
 It is a fractal which construction starts form a triple school in the age of the computer. BasicBooks, 10
 East 53rd St., New York, NY 10022-5299.
circles , , , each tangent to the other two. It [2]. Robová, J. (2012). Výzkumy vlivu některých typů
follows a filling in circles, each tangent to another technologií na vědomosti a dovednosti žáků v
three. A part of the solution is presented in Fig. 10. matematice. Scientia in educatione, 3(2), 79-106.
 [3]. Kalaš, R. I. (2010). Premeny školy v digitálnom
 veku. DidInfo 2010.
 [4]. Hejný, M. (1989). Teória vyučovania matematiky. Dl.
 2. SPN.
 [5]. Fulier, J. (2018). Niekoľko poznámok o aspektoch
 vizualizácie v matematickom vzdelávaní. Acta
 Mathematica Nitriensia, 4(1), 24-38.
 [6]. Huvent, G. (2008). Sangaku: le mystère des énigmes
 géométriques japonaises. Dunod.
 [7]. Fukagawa, H., & Rothman, T. (2008). Sacred
 mathematics: Japanese temple geometry. Princeton
 University Press.
 [8]. Rothman, T., & Fukagawa, H. (1998). Japanese
 temple geometry. Scientific American, 278(5), 84-91.
 [9]. Bartsch, H. J. (2014). Handbook of mathematical
 formulas. Academic press.
 [10]. Švrček, F. (2005). O posloupnostech kružnic s
 Figure 10. A part of Apollonius’ net vnějším dotykem. Rozhledy matematicko-
 fyzikální, 80(4), 5-14.
6. Conclusion [11]. Ogilvy, C. S. (1990). Excursions in geometry.
 Courier Corporation.
 Visual communication in mathematics is a specific
 [12]. Andrade Jr, J. S., Herrmann, H. J., Andrade, R. F., &
phenomenon that is based on the visualization of Da Silva, L. R. (2005). Apollonian networks:
mathematical concepts and situations. More Simultaneously scale-free, small world, Euclidean,
precisely, these are various geometric representations space filling, and with matching graphs. Physical
of mathematical concepts, which are useful to use review letters, 94(1), 018702.
wherever it is possible, purposeful, and functional [13]. Polák, J. (2016). Didaktika matematiky: jak učit
[13]. matematiku zajímavě a užitečně. Obecná didaktika
 The concept of the visualization of learning matematiky. II. část. Fraus.
materials stimulates the educational process and [14]. Semenikhina, O., Kudrina, O., Koriakin, O.,
requires teachers’ professional activity. The well- Ponomarenko, L., Korinna, H., & Krasilov, A.
 (2020). The Formation of Skills to Visualize by the
mastering of dynamical visualizations by computer
 Tools of Computer Visualization. TEM Journal, 9(4),
requires understanding of a technological 1704.
background of software in solving mathematical task [15]. Velichová, D. (2011). Interactive maths with
[14]. GeoGebra. International Journal of Emerging
 It is important to provide software that allows Technologies in Learning (iJET), 6(2011).
activities to the development of students’ competence
to work with various representations of mathematical
objects. GeoGebra is one of these didactic tools [15].
 Based on the ideas above, we used the solution of
the selected geometric sangaku problem to the
demonstration of the efficiency and benefits of the
application of the dynamical geometry software.

TEM Journal – Volume 10 / Number 2 / 2021. 545